The associative property isn’t just another dusty mathematical concept relegated to textbooks. It’s a fundamental principle that permeates the very fabric of mathematics, acting as a master key that unlocks order and consistency within complex systems. Imagine a world where rearranging numbers in an equation drastically altered the answer – chaos would reign! The associative property ensures this mathematical nightmare never unfolds. But what exactly is this enigmatic property, and how does it weave its magic?
At its core, the associative property dictates how grouping affects the outcome of operations. In simpler terms, it doesn’t matter how you huddle your numbers together as long as you perform the operations (addition, multiplication, etc.) in the right order, the final answer remains unchanged. Think of it like rearranging ingredients in a recipe – as long as you add the flour before the baking powder, the end result (hopefully!) will still be a delicious cake.
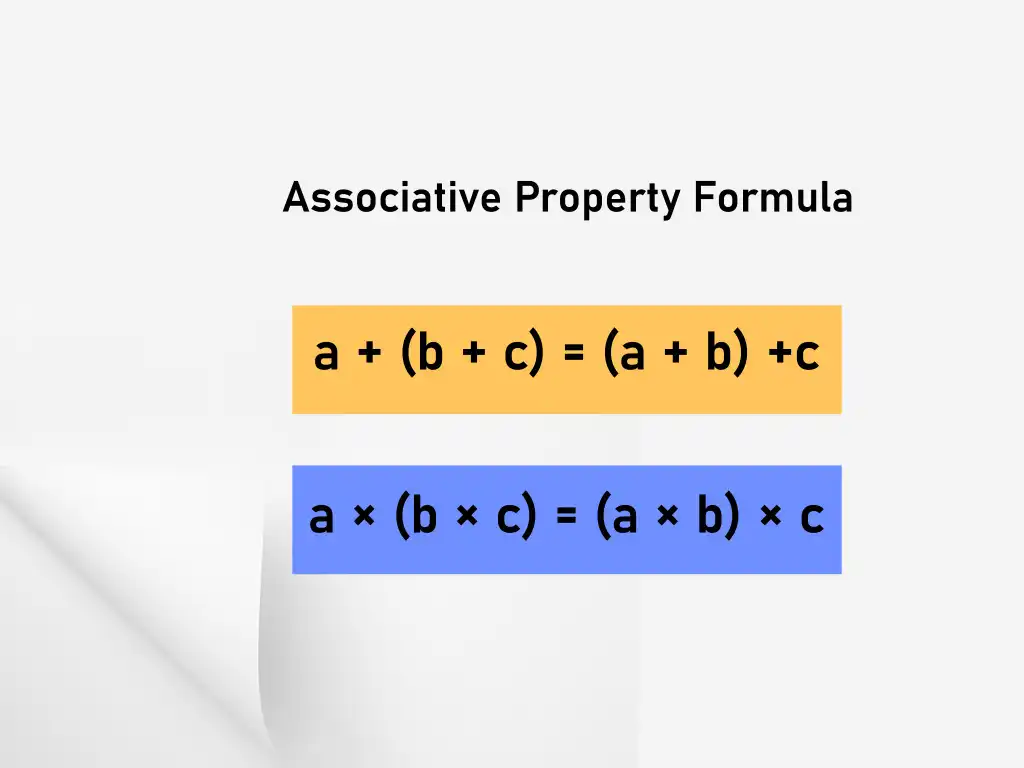
Here’s where things get a little more technical. The mathematical representation for the associative property in addition looks like this:
(a + b) + c = a + (b + c)
This equation simply states that adding “a” to the sum of “b” and “c” yields the same result as adding “b” to the sum of “a” and “c” – as long as we’re sticking to addition throughout. But the magic of the associative property extends beyond mere addition. It applies to multiplication as well, and under specific conditions, even subtraction. However, it’s important to remember that this property only holds true for specific operations within a number system; try rearranging exponents in an equation, and you might end up with a mathematical monster on your hands!
The Associative Property in Action: From Balancing Equations to Balancing Your Budget
The associative property isn’t just an abstract concept confined to the realm of mathematicians. It infiltrates various aspects of our lives, even if we don’t always recognize its presence. Let’s delve into a few intriguing examples:
- Balancing Chemical Equations: When we balance the intricate dance of reactants and products in a chemical equation, the associative property allows us to rearrange the coefficients (those multiplying factors before a chemical formula) without disrupting the overall reaction. Imagine a recipe for a chemical reaction – the associative property ensures that rearranging the ingredients (reactants) doesn’t alter the final product.
- Simplifying Expressions: Ever encountered a mathematical expression that resembles a tangled mess of numbers and operations? The associative property comes to the rescue! By regrouping terms based on this property, we can untangle the expression and simplify it, revealing the hidden answer within.
- Computer Science and Programming: The world of computers and coding heavily relies on the associative property. It ensures that the order of evaluation for certain operations doesn’t affect the final outcome, as long as the hierarchy of operations (think of it as the pecking order of mathematical calculations) is respected.
- Financial Calculations: When calculating the wonders of compound interest, the associative property allows us to group interest earned over different periods without altering the total amount we’ll end up with. After all, the final interest earned depends on the overall principal and interest rate, not necessarily on the specific way the interest is calculated for each period.