The commutative property, a cornerstone of mathematics, weaves a magical spell on the order of numbers in addition and multiplication. It proclaims that the sequence in which we add or multiply numbers holds no dominion over the final outcome, as long as the sacred essence of the numbers themselves remains unchanged. This seemingly innocuous principle forms the bedrock of a plethora of mathematical operations, streamlining calculations and fostering a deeper comprehension of the enigmatic relationships between numbers.
Illuminating the Commutative Property Through Practical Examples
Let’s embark on a voyage of discovery through the realm of examples to illuminate the essence of the commutative property.
Addition: A Paradigm of Order Independence
Consider the expression 3 + 5. The commutative property empowers us to rearrange the numbers in a whimsical dance, yet the sum remains steadfast:
- 3 + 5 = 8
- 5 + 3 = 8
In both scenarios, irrespective of whether we add 3 to 5 or 5 to 3, the answer remains a harmonious 8. This beautifully exemplifies the commutative property of addition, expressed in the following elegant formula:
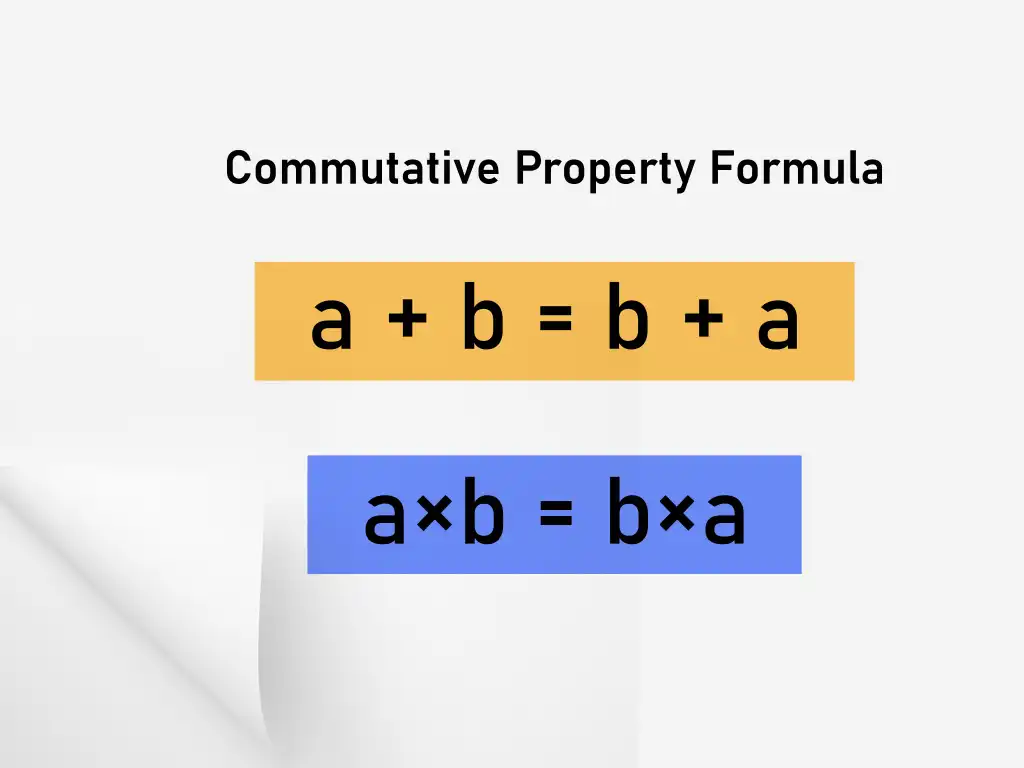
(a + b) = (b + a)
Multiplication: Another Realm of Orderly Freedom
The commutative property, like a benevolent deity, extends its dominion to the realm of multiplication as well. Take the expression 2 x 4. We can rearrange the numbers while maintaining the product:
- 2 x 4 = 8
- 4 x 2 = 8
Once again, the order of multiplication exerts no influence on the outcome (8). This beautifully demonstrates the commutative property of multiplication, encapsulated in the following formula:
(a x b) = (b x a)
These examples illuminate the power of the commutative property. It allows us to conquer expressions with finesse by focusing on individual calculations within parentheses before uniting them. This elegant strategy streamlines the process and minimizes the potential for errors, allowing us to solve problems with the grace of a seasoned mathematician.
The Commutative Property: A Ubiquitous Force in Mathematics
The commutative property transcends the boundaries of simple addition and multiplication, weaving its magic throughout the tapestry of mathematical operations and concepts. Here, we delve into some of its noteworthy applications:
-
Evaluating Expressions with Strategic Maneuvers: When grappling with complex expressions brimming with multiple operations, the commutative property empowers us to strategically rearrange terms, facilitating a smoother evaluation process. We can prioritize calculations within parentheses without jeopardizing the overall outcome, akin to a general marshalling troops in a grand battle.
-
The Distributive Property: A Blood Relative: The distributive property, another cornerstone of mathematics, is inextricably linked to the commutative property. It establishes a connection between the multiplication of a sum or difference and the individual terms nestled within the parentheses. This intricate relationship underscores the interconnectedness of these fundamental properties, like the branches of a mighty oak tree.
-
Abstract Algebra: A Realm of Orderly Structures: The commutative property plays a pivotal role in abstract algebra, particularly in the study of groups and rings. These abstract structures hinge on the ability to combine elements while preserving specific relationships, a feat guaranteed by the commutative property. It forms the foundation upon which these structures are built, much like the laws of physics govern the movements of celestial bodies.
Beyond Arithmetic: The Commutative Property’s Broader Influence
The influence of the commutative property extends far beyond the realm of arithmetic, finding applications in diverse disciplines:
-
Logic: The Orderly World of Propositions: In propositional logic, the commutative property governs the order of operations within logical expressions. It ensures that the truth value of a compound statement remains unchanged regardless of how we group parentheses, ensuring clear and unambiguous communication.
-
Computer Science: The Efficiency of Order: In the intricate world of computer programming, the commutative property dictates the order of evaluation for certain operators within an expression. This knowledge is paramount for crafting efficient and predictable code, akin to the well-oiled gears of a machine working in perfect harmony.
The Commutative Property: A Cornerstone for Learning Math
Mastering the commutative property equips students with a powerful arsenal of tools:
-
Problem-Solving Prowess: By comprehending how order affects calculations, students develop the ability to approach problems with greater flexibility. They can strategically choose the most efficient method to solve an expression based on the numbers involved, much like a skilled chess player formulating winning strategies.
-
Conquering Complexity: The commutative property empowers students to break down complex expressions into manageable chunks by strategically employing parentheses. This invaluable skill simplifies calculations and reduces the likelihood of errors, fostering a sense of accomplishment and confidence in their mathematical abilities.
-
Building a Foundation for Mathematical Exploration: The commutative property underpins a vast array of advanced mathematical concepts. A solid grasp of this principle lays the groundwork for further exploration in algebra, calculus